Publications and Preprints
5. Lagrangian Translating Solitons and Special Lagrangians in \(\mathbb{C}^m\) with Symmetries
Wei-Bo Su, Albert Wood
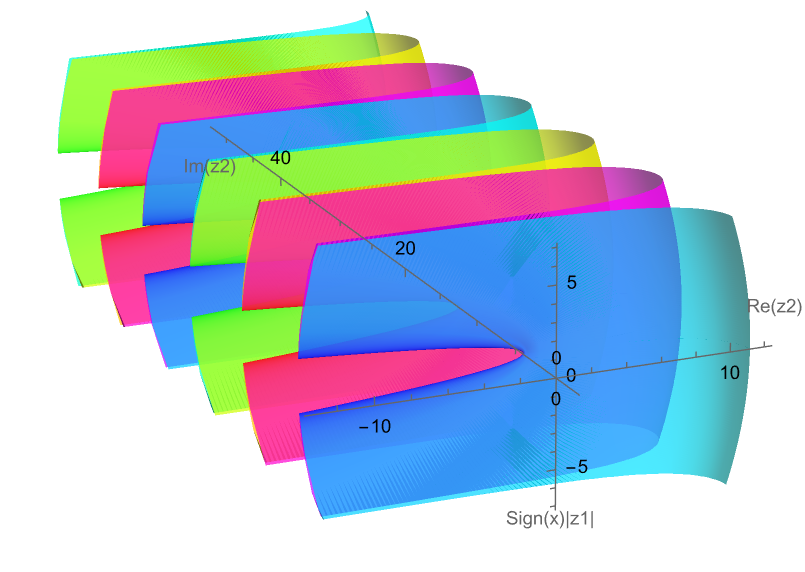
Abstract: We construct novel families of exact immersed and embedded Lagrangian translating solitons and special Lagrangian submanifolds in \(\mathbb{C}^m\) that are invariant under the action of various admissible compact subgroups \(G\leq \text{SU}(m-1)\) with cohomogeneity-two. These examples are obtained via an Ansatz generalising a construction of Castro-Lerma in \(\mathbb{C}^2\). We give explicit examples of admissible group actions, including a full classification for \(G\) simple. We also describe novel Lagrangian translators symmetric with respect to non-compact subgroups of the affine special unitary group \(\text{SU}(m)\ltimes \mathbb{C}^m\), including cohomogeneity-one examples.
4. Infinite-Time Singularities of the Lagrangian Mean Curvature Flow
Wei-Bo Su, Chung-Jun Tsai, Albert Wood
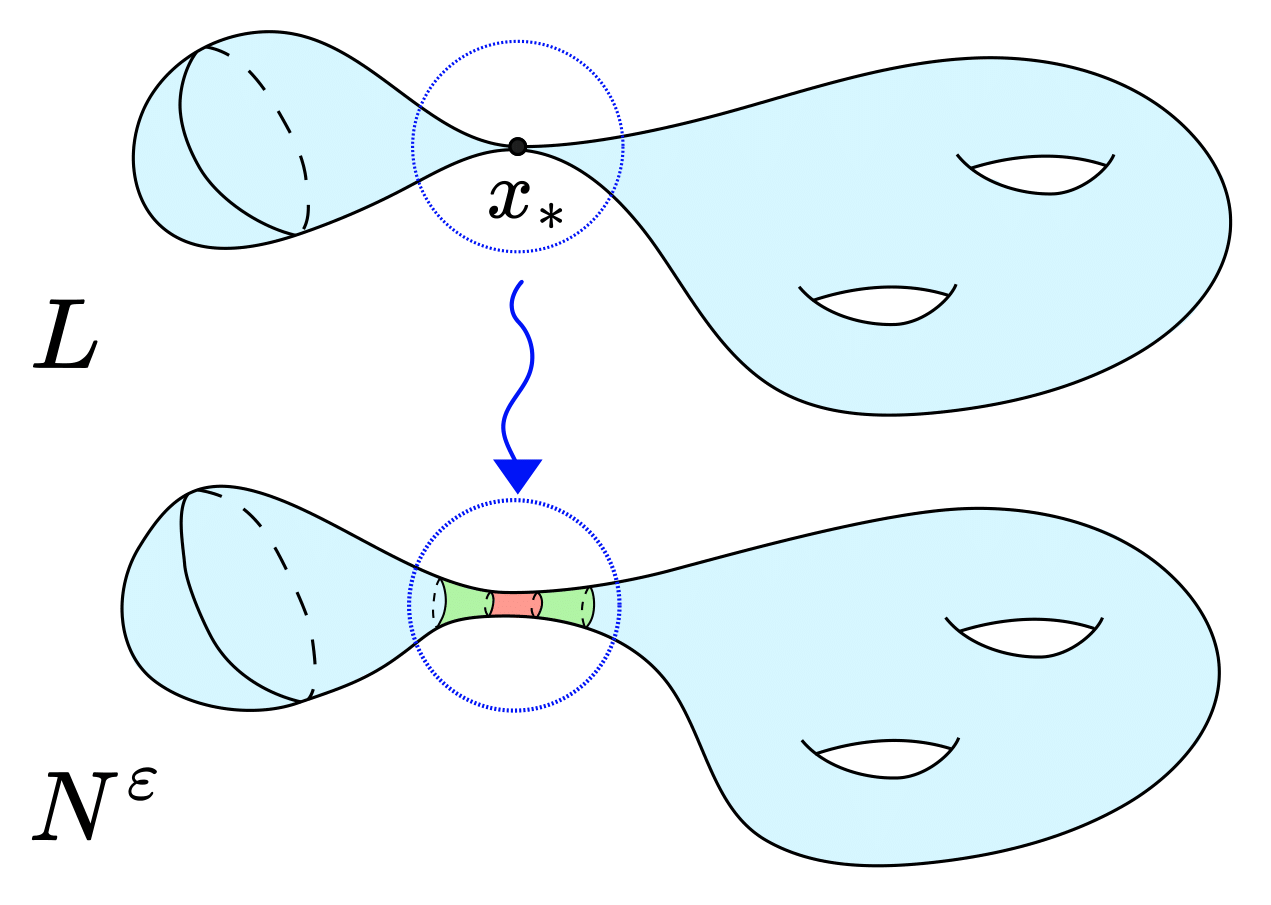
Abstract: We exhibit examples of Lagrangian mean curvature flow which exist and are embedded for all time, but form an infinite-time singularity and converge to an immersed special Lagrangian as \(t\rightarrow \infty\) This result shows that infinite-time singularities can form in the Thomas--Yau `semi-stable' situation. Our work is a parabolic analogue of the results of Dominic Joyce and Yng-Ing Lee regarding desingularisation of special Lagrangians with conical singularities. The gluing construction that we employ is inspired by the work of Simon Brendle and Nikolaos Kapouleas regarding ancient solutions of the Ricci flow.
3. Cohomogeneity-One Lagrangian Mean Curvature Flow
Math. Ann. 391 Vol. 7 (2025), Jesse Madnick, Albert Wood
Video Lectures: S02T02 Albert Wood
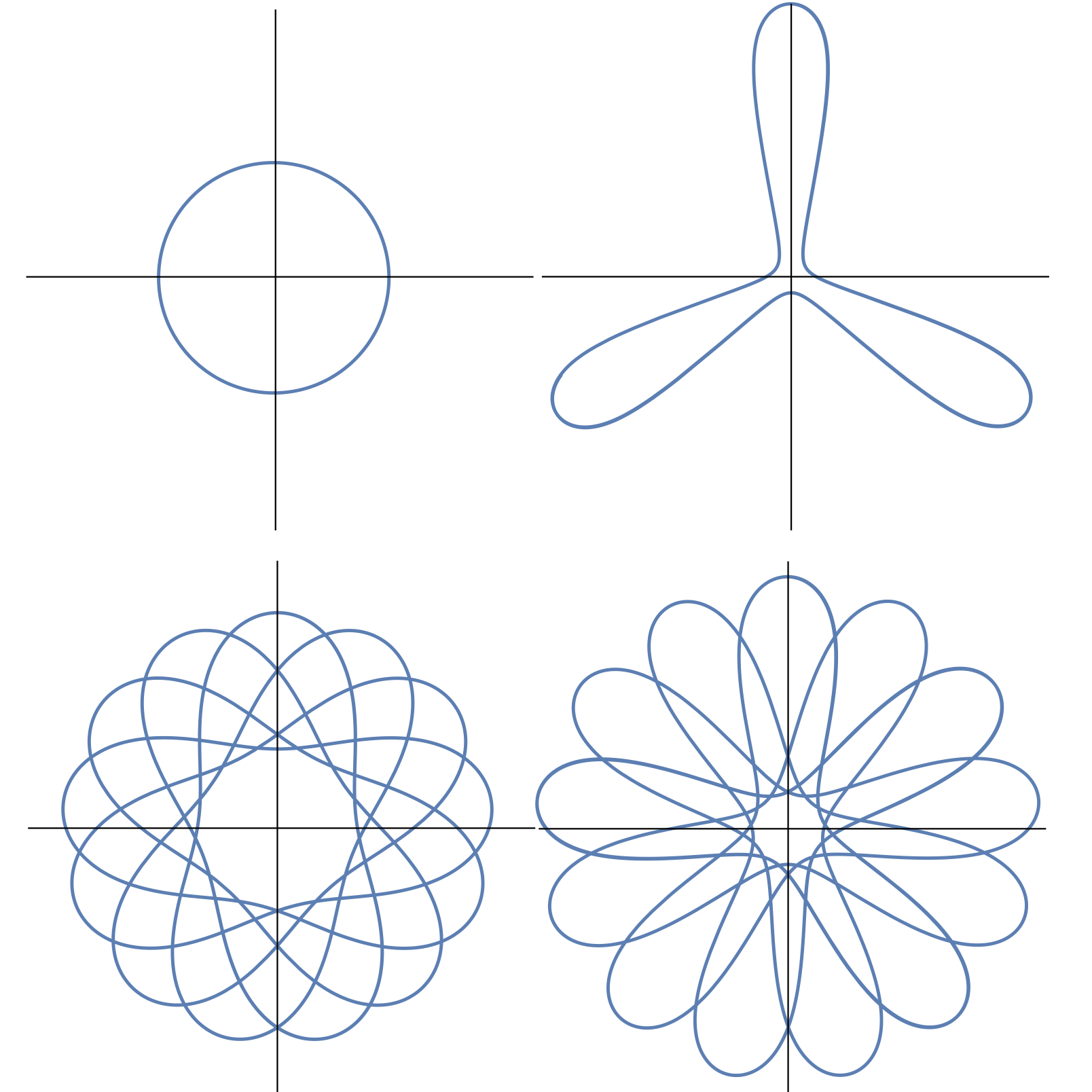
Abstract: We study mean curvature flow of Lagrangians in \(\mathbb{C}^n\) that are cohomogeneity-one under a compact Lie group \(G\leq SU(n)\) acting linearly on \(\mathbb{C}^n\). Each such Lagrangian necessarily lies in a level set \(\mu^{-1}(\xi)\) of the standard moment map \(\mu:\mathbb{C}^n\rightarrow \mathfrak{g}^*\), and mean curvature flow preserves this containment. We classify all cohomogeneity-one self-similarly shrinking, expanding and translating solutions to the flow, as well as cohomogeneity-one smooth special Lagrangians lying in \(\mu^{-1}(0)\). Restricting to the case of almost-calibrated flows in the zero level set \(\mu^{-1}(0)\), we classify finite-time singularities, explicitly describing the Type I and Type II blowup models. Finally, given any cohomogeneity-one special Lagrangian submanifold in \(\mu^{-1}(0)\), we show it occurs as the Type II blowup model of a Lagrangian MCF singularity, thereby providing infinitely many previously unobserved singularity models. Throughout, we give explicit examples of suitable group actions, including a complete list in the case of \(G\) simple.
2. Singularities of Equivariant Lagrangian Mean Curvature Flow
Comm. Anal. Geom. 37 Vol. 7 (2024), Albert Wood
Video Lectures: [1st GAF] Albert Wood: Singularities of Lagrangian Mean Curvature Flow
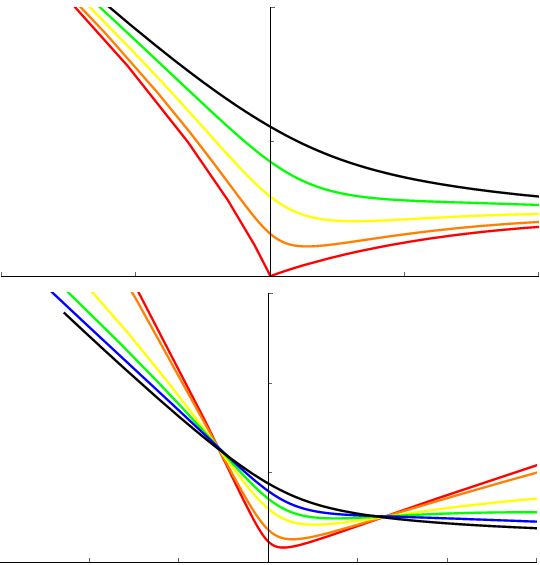
Abstract: We study almost-calibrated, \(O(n)\)-equivariant Lagrangian mean curvature flow in \(\mathbb{C}^n\), and prove structural theorems about the Type I and Type II blowups of finite-time singularities. In particular, we prove that any Type I blowup of such a flow must be a special Lagrangian pair of transversely intersecting planes, any Type II blowup must be the Lawlor neck with the same asymptotes, and these blowups are independent of the choice of rescaling. We also give a partial classification of when singularities occur in the equivariant case, and examine the intermediate scales between the Type I and Type II models.
1. Lagrangian Mean Curvature Flow with Boundary
Calc. Var. 61, 106 (2022), Chris Evans, Ben Lambert, Albert Wood
Video Lectures: [3rd GAF MC] Albert Wood: Lagrangian Mean Curvature Flow with Boundary
Abstract: We introduce Lagrangian mean curvature flow with boundary in Calabi-Yau manifolds by defining a natural mixed Dirichlet-Neumann boundary condition, and prove that under this flow, the Lagrangian condition is preserved. We also study in detail the flow of equivariant Lagrangian discs with boundary on the Lawlor neck and the self-shrinking Clifford torus, and demonstrate long-time existence and convergence of the flow in the first instance and of the rescaled flow in the second.
PhD Thesis
Singularities of Lagrangian Mean Curvature Flow
PhD Thesis, completed as part of the PhD Degree at University College London
Supervised by Prof. Felix Schulze
I was awarded my PhD in September 2022 from University College London. The topic of my thesis is Lagrangian mean curvature flow, focusing on singular behaviour and boundary conditions for the flow. When writing my thesis, I aimed to create a resource suitable for newcomers learning the subject of Lagrangian mean curvature flow for the first time; in particular I provided motivating material from the theory of mean curvature flow as well as an exposition of the original proof by Smoczyk of the preservation of the Lagrangian condition. If you would like to read it or view the abstract, please click on the title above.
Short Research Projects
4. Machine Learning Calabi-Yau Metrics
Group Project, completed as part of LOGML Summer School 2024, with Marek Černý, Phillipp Dahlinger, Heath Pearson, Tom Walker
Supervised by Dr. Yidi Qi
Abstract: We investigated the problem of discovering new Calabi-Yau metrics on hypersurfaces of complex projective space using machine learning techniques. We represented the problem of finding a Calabi-Yau metric on a hypersurface as a supervised learning problem, tested three different types of neural network (bihomogeneous, spectral and \(U(1)\)-invariant networks) and compared the errors for the resulting metric.
3. On Belyi Functions and their Applications
Short Project, completed as part of the LSGNT Doctoral School
Supervised by Prof. Andrew Granville, Dr. Davoud Cheraghi
Abstract: We examine the application of the theory of dessins d'enfant to the problem of bounding the difference of coprime polynomials. The existence of a sharp bound is equated to the existence of a particular multigraph, which is tractable with basic combinatorial techniques.
2. Collapse in Riemannian Geometry
Short Project, completed as part of the LSGNT Doctoral School, with Fabian Lehmann, Jenny Swinson
Supervised by Prof. Mark Haskins, Prof. Jason Lotay
Abstract: We give an exposition on the phenomenon of collapsing Riemannian metrics with bounded sectional curvature, focusing on the important contributions of Cheeger-Gromov and Foscolo.
1. Combinatorial Applications of Szemerédi's Regularity Lemma
Master's Thesis, completed as part of the MMath Degree at University of Oxford
Supervised by Prof. Ben Green
Abstract: Szemerédi’s Regularity Lemma is a result of extremal graph theory, which roughly states that all graphs can be partitioned such that the edges between the partition sets appear to have been generated at random. It therefore helps us when the result we are trying to prove is expected to be true for random graphs. In this dissertation, we cover some applications of Szemerédi’s Regularity Lemma to number theory and additive combinatorics.
